Introduction to Laplace Transform Calculator with Steps
The Laplace calculator is an online tool that is used to transform integral functions using the Laplace method. This tool helps to change the complex variable integral function to the real variable function in a fraction of a second.

The Laplace transform is a mathematical operation that transforms a function of time (often a function of a real variable t representing time) into a function of a complex variables.This transformation is particularly useful in solving linear differential equations, especially those with constant coefficients, by converting them into algebraic equations.
The Laplace Transform Calculator is a versatile tool designed to compute the Laplace transform of a given function efficiently.
Additionally, A Laplace transform calculator is a used to compute the Laplace transform of a given function. Further, if you would like to determine the solving of complex equations and optimize the differential equation along with boundary problems in less than a minute with no error, you can use our laplacian calculator with steps. Our calculator is a valuable tool for students, researchers, and professionals for doing research work, making reports, and used in telecommunication and scientific fields, and other applications of daily life.
What is Laplace Transform?
Laplace transform is defined as an integral function that is the multiple of the complex variable of the differential equation. It is a mathematical technique that allows you to transform a function into a new simple form in which you can easily evaluate integral problems.
The Laplace transform is a mathematical operation that transforms a function of time (or other independent variable) into a function of a complex variable. It is particularly useful in solving linear differential equations, especially those with constant coefficients.
The Laplace transform is particularly useful in analyzing linear time-invariant systems and solving differential equations with initial conditions. Additionally, if you would like to find z transform series of complex variables, you can use our z transform solver. Our calculator also evaluate a series that belongs to the real number or complex number into a discrete-time signal that can change into the frequency domain in real time.
Formula used by Laplace Calculator
The formula used by our laplace transformation calculator is given as,
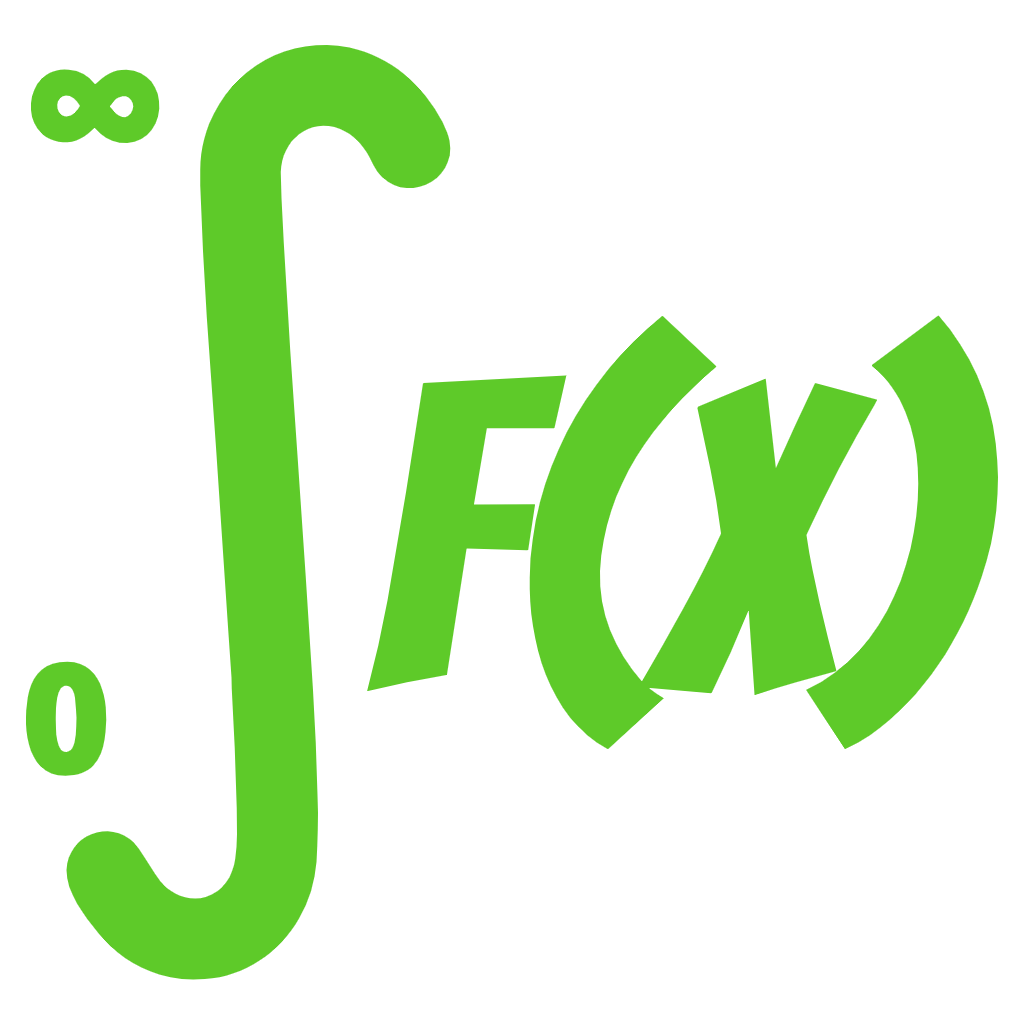
$$ \mathcal{L} {f(t)} \;=\; \int_{0}^{\infty} e^{-st} f(t) dt $$
In the formula L is the notation of Laplace
where,
f(t) is the Laplace function
e-st is the complex function
dt is the integration variable
0 to is the lower and upper limits.
Working Procedure in Piecewise Laplace Transform Calculator
The Laplace transform piecewise calculator followed the procedure of Laplace rules which are used to solve different combinations of differential equations. This method is used when a complex variable differential function changes real values.
When you give input to our tool, it will check the function's behavior and then apply the exact rules of laplace which are required to get the result of a given function. Then it adds in the solution of the given function.
Resultantly, it will give results of ordinary differential equations into simple polynomial equations according to your functions.
This transformation simplifies the analysis and solution of linear differential equations, especially in the engineering field and control theory in technology.If you're interested in exploring related mathematical tools, you might also find our fourier sine series calculator useful for analyzing periodic functions and decomposing them into their frequency components.
Let us see an example of Laplace transform using our Laplace transform step function calculator.
Solved Example of Laplace Transformation Calculator
An example of the Laplace transform function which is solved in our piecewise Laplace transform calculator is,
Example:
Find the Laplace transform of e-5t + cost2 (2t).
Solution:
First of all, write the real variable functions.
$$ f (t) \;=\; e^{-5t} $$
$$ g (t) \;=\; cos^{2} 2t $$
Using the Laplace notation,
$$ \mathcal{L} {f(t) + g(t)} \;=\; \mathcal{L} \Biggr[e^{-5t} + cos^2 (2t) \Biggr] $$
Use the Laplace linearity property and apply the notation separately,
$$ \mathcal{L} \Biggr[e^{-5t} + cos^2 (2t) \Biggr] \;=\; \mathcal{L} e^{-5t} + \mathcal{L} \Biggr[ cos^2 (2t) \Biggr] $$
Transform the above functions using the Laplace table,
$$ \mathcal{L} \Biggr[e^{-5t} \Biggr] \;=\; \frac{1}{s + 5} $$
$$ \mathcal{L} \Biggr[ cos^2 (2t) \Biggr] \;=\; \frac{1}{s} + \frac{s}{2(s^2 + 16)} $$
Write the calculated values with the given function,
$$ \mathcal{L} \Biggr[ e^{-5t} + cos^2 (2t) \Biggr] \;=\; \frac{1}{s+5} + \frac{1}{2s} + \frac{s}{2(s^2 + 16)} $$
Thus it is the final solution of our function with specific limits.To further analyze or manipulate this expression, including finding the inverse Laplace transform, simplifying, or performing additional calculations, you can use our inverse laplace transform calculator with steps. This calculator is specifically designed to handle Laplace transforms and provides efficient solutions for various functions.
How to Use the Laplace Transform Piecewise Calculator
Laplace transform calculator with steps is simply a design tool which means it can be accessible for everyone if you follow some instructions. These steps are:
- Enter the function in the respective field in the format of f(t)or g(t).
- Review your function before pressing the calculate button.
- Click on the “ Calculate” button to get the solution of the given function.
The Result Obtained from Laplace Transform Step Function Calculator
The Laplace calculator will give the required function result immediately after you enter the function in it.
It may include as:
By incorporating these features, our Laplace Transform Calculator offers users a seamless experience in obtaining accurate results and gaining insights into the transformed functions. For further mathematical analysis and computations, you can use our integral finder. Our calculator also makes the calculations faster and gives the required solution of integral function in a fraction of a second.
- Solution will appear in the box.
- Click on the plot box to get a graphical representation of the given function
- Click on the “Recalculate” button for more practice.
Benefits of Using the Laplace Calculator with Steps
Laplace Transform calculator provides many benefits because it gives periodic functions from complex ones into simplified forms without any struggle. These may include as:
Utilizing our Laplace Transform Calculator empowers users to tackle complex problems with ease, whether they're solving differential equations or analyzing dynamic systems. For access to this calculator and many more, you can explore our comprehensive collection of calculators on the All Calculators page.
- Our laplace transformation calculator saves time and gives results of the particular function immediately.
- It is a user-friendly device so anyone can use it.
- Our Laplace transform piecewise calculator has an advanced server of Laplace rules that help you always to give accurate results
- It is essential in engineering, physics, control theory, complex differential equations solution
- The Laplace transform step function calculator is very useful to solve differential equations which are mostly used to transform the analysis of the data values. This online tool is the best solver for students and researchers.
Conclusion
Laplace calculator provides solutions for different kinds of differential equations when the initial condition is zero.
Our Laplace transform calculator provides help in scientific and engineering fields because these fields need faster calculations of complex functions. With that, you can check out the fourier integral calculator for complex functions of frequency.