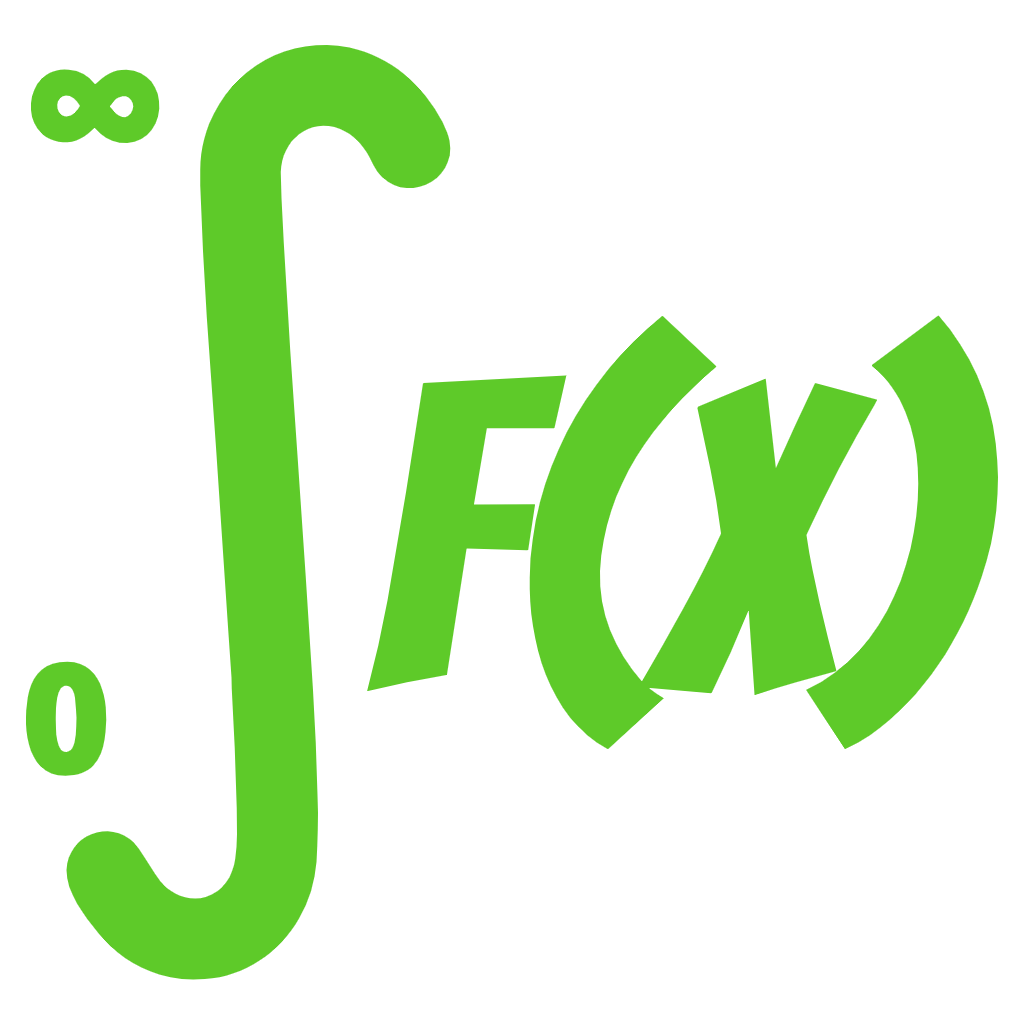
Introduction to the Improper Integral Calculator
An improper integral convergence calculator is a free online tool for finding improper integral functions. It is designed to solve complex improper integrands that have one or two infinite limit values.

This convergent integral calculator is very beneficial for students, teachers, or researchers who want to improve their knowledge of improper integral concepts efficiently without any struggle.
So improper integral calculator help to solve complex integral that have one or two infinite limit values. Addionally, if you would need to find an area enclosed in a graph in a bounded region. you can use a our definite integration solver for compute definite Integration of single-variable functions.
What is Improper Integral?
Improper integral is defined as an area under the curve that may have both or one infinite upper or lower limits or may its integrand tend to infinity. It is the type of definite integral but in a discontinuity form of interval. It may converge or diverge depending on the result of the improper integral questions, if it gives a finite answer then it shows convergence otherwise it diverges.
Improper integrals arise when the function being integrated is not defined or is unbounded at one or both endpoints of the interval of integration. Improper integrals can also arise if the interval of integration is infinite. In such cases, limits are used to define the integral. You can use our curve area calculator to visualize and compute the area under curves, especially when dealing with improper integrals.
Formula Behind the Integral Convergence Calculator
The improper integral formula has two types that are given below. The formula used by our improper integral calculator is,
-
One-Sided Infinite Limit
Let f(x) be the function of integral that is continuous on an interval where one limit (the upper or lower) value is infinite.
If f(x) is continuous on a, ∞ the improper integral of f over a,∞ will be,
$$ \int_{a}^{\infty} f(x) dx \;=\; \lim_{R \to \infty} \int_{a}^{R} f(x)dx $$
If f(x) is continuous on -∞,b the improper integral of f over -∞,b will be,
$$ \int_{-\infty}^{b} f(x)dx \;=\; \lim_{R \to -\infty} \int_{R}^{b} f(x) dx $$
-
Two-Sided Infinite Limits
Let f(x) be an integral function that is continuous and both limits (the upper and the lower) limit are infinity. It converges when both functions converge because we split both infinite limits into two functions using a constant number of limits.
$$ \int_{-\infty}^{\infty} f(x)dx \;=\; \int_{-infty}^{a} f(x)dx + \int_{a}^{\infty} f(x) dx $$
Addionally, for computations involving double integrals, you can utilize our multiple integrals calculator for efficient and precise solutions. Our calculator can also solve all definite double integral functions of a given function when you give input along with the upper and lower limits of x and y coordinates respectively.
Working Process of Convergent Integral Calculator
The improper integral calculator uses a simple working procedure for the solution of one side infinite limit or even for a complex infinite integral function. This tool has advanced algorithmic where all definite integral principles are set up in its server.
When you give input in the convergent or divergent integral calculator, it would analyze whether the given question is related to a one-side infinite limit or a two-side infinite limit. After recognizing the type of function it applies the limit condition and then solves the given problem with a definite integral method.
After integration, the integral divergence calculator applies the upper and the lower limits and gets the solution to the improper integral question. If the limit value solution is finite then it converges otherwise if infinite values get then diverge.
Additionally, for evaluating indefinite integrals, you can use our infinite integrals calculator to find antiderivatives and explore the family of functions corresponding to a given function. Also our calculator offers a convenient way to understand the behavior of functions and the relationships between different functions through their antiderivatives, aiding in various mathematical analyses and problem-solving tasks.
Solved Example of Improper Integral
An example of the improper integral problem is given to let you know how to solve such problems manually. These problems can be solved by using the improper integral calculator but it is also important to know each step so, here’s an example,
Example:
Determine the following:
$$ \int_{0}^{\infty} (1+2x) e^{-x} dx $$
Solution:
$$ \int_{0}^{\infty} (1+ 2x) e^{-x} dx \;=\; \lim_{t \to \infty} \int_{0}^{t} (1+2x) e^{-x} dx $$
$$ u\;=\; 1+ 2x \to \;du\;=\; 2dx $$
$$ dv\;=\; e^{-x} dx \to \;v\;=\; -e^{-x} $$
$$ \int (1+2x) e^{-x} dx \;=\; -(1+2x) e^{-x} + 2 \int e^{-x} dx \;=\; -(1+2x) e^{-x} - 2e^{-x} + c \;=\; -(3+2x) e^{-x} + c $$
$$ \int_{0}^{\infty} (1+2x) e^{-x} dx \;=\; \lim_{t \to \infty} \biggr(- (3+2x)e^{-x} \biggr) \biggr|_{0}^{t} \;=\; \lim_{t \to \infty} (3-(3+2t) e^{-t}) $$
$$ \int_{0}^{\infty} (1+2x) e^{-x} dx \;=\; \lim{t \to \infty} 3 - \lim_{t \to \infty} \frac{3+2t}{e^t} \;=\; 3- \lim_{t \to \infty} \frac{2}{e^t} \;=\; 3 - 0 \;=\; 3 $$
Thus it is the final solution of the given limits function. Additionally, if you would like to determine the displacement of triple integral function in a defined region in a three-dimensional space. You can use our calculate triple integral. Our calculator is particularly useful in situations where performing the integration manually is complex or time-consuming.
How to Use an Improper Integral Calculator?
This improper integral convergence calculator will give results instantly if you follow some simple steps for complex improper integral with undefined limits. These steps are:
For more complex integration problems involving curves in vector fields, use may utilize our line integral along curve calculator. Our tool facilitates the computation of line integrals, aiding in various applications in physics, engineering, and mathematics.
- Put your particular integral problem as an input in its respective box.
- Now, select the independent variable in terms of which function is integrated
- Add the upper and lower limit in its relevant fields
- Review improper functions before clicking on the calculate button.
- Press the “Calculate” button for desired results.
- You can click on the “Recalculate” button for further calculation
Outcome from Convergent or Divergent Integral Calculator
An convergent integral calculator gives you a solution to your given integral function in a fraction of a second. You can get other extra options with the result that enable you to understand the improper integration concept easily. It may include as:
-
Result Field
Result Option gives you a solution to your given improper integral problems
-
Possible Steps
It will give improper integration solutions in step by step process
-
Plot
Plot sketch a graph according to the solution of the improper integral problem that shows function converges or diverges.
-
Definite Integral
It gives the solution of the definite integral of the given function
For further analysis and computation of integrals, you can explore our integrate calculator. Our comprehensive tool offers solutions for both definite and indefinite integrals, empowering users to tackle a wide range of mathematical problems with ease
Advantages of Using the Integral Divergence Calculator
You can get maximum benefits while you using it to evaluate complicated integral problems in our improper integral calculator. These advantages are
Additionally, use can use our finding antiderivatives calculator to find antiderivatives of functions, providing further insights into the relationship between integration and differentiation. Our calculator also offers a user-friendly interface and accurate results, making it a valuable tool for studying and applying integral calculus concepts in various mathematical contexts.
- It keeps you away from doing long-form calculations of complex improper integral questions
- The convergent or divergent integral calculator is a free tool that does not charge any fee for evaluation.
- You can use our calculator to solve unlimited examples of infinite integral functions
- It has a user-friendly interface so you can use it for improper integral functions easily.
- It provides you with a graphical representation of improper functions to get better clarity of its convergence and divergence
- You can use our calculator from your mobile, computer laptop through the internet
- It provides you with accurate solutions of improper integral with steps
- Our improper integral calculator gives a solution within a minute when you use it for improper integrals( whether a given problem is complex or not).