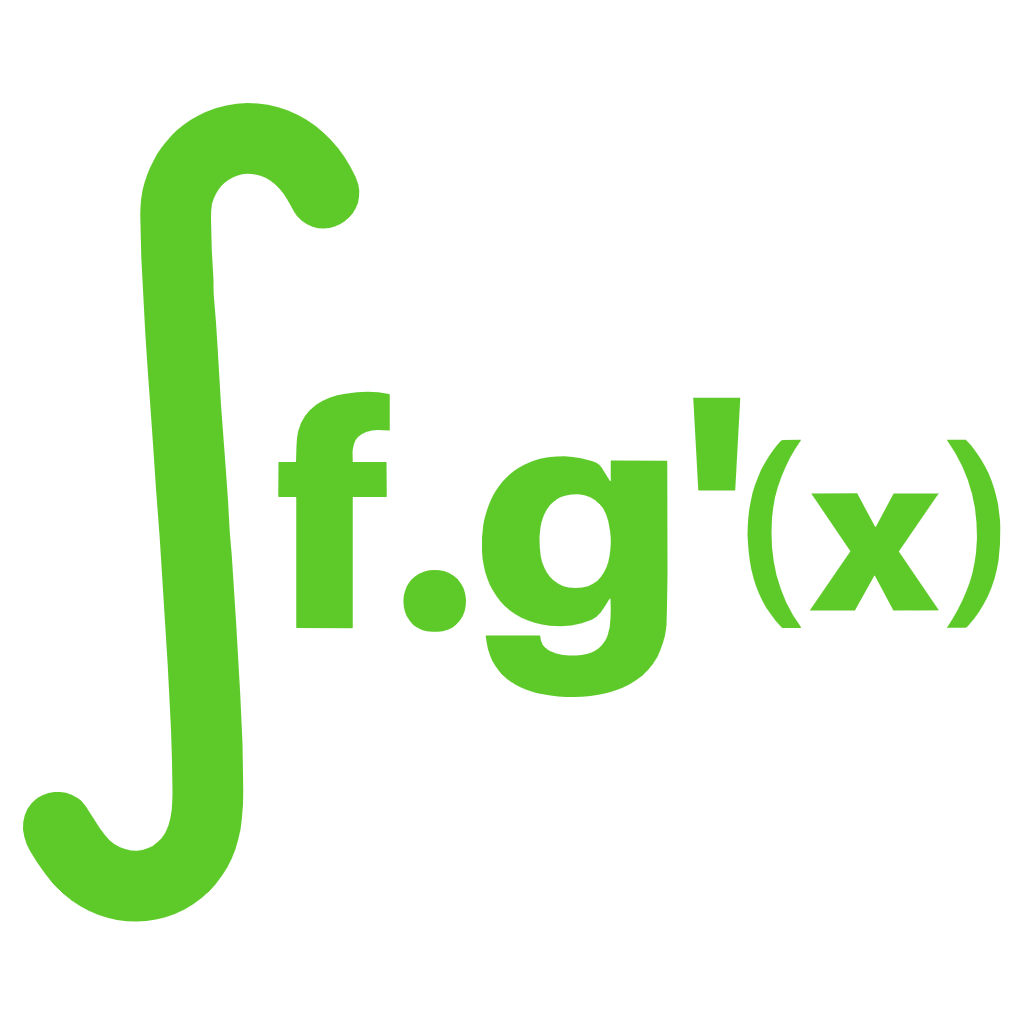Introduction to Integration by Parts Calculator
Integral by parts calculator is a free online tool that is used to find the antiderivative for the given function. It helps you to easily determine the special type of integration which is a product of two functions.

In this ibp calculator, you can get a simplified process for the evaluation of both definite and indefinite integral functions using the by parts method or rule.
The calculator provides step-by-step solutions, making it easier for users to understand the process and learn from it. It is an invaluable tool for students, professionals, and anyone dealing with integration problems in mathematics, physics, engineering, and other fields.
Additionally, our cacluator is designed to simplify the process of solving integration problems using the integration by parts method. Moreover, if you would to to find integral problems of rational algebraic expression, you can use our partial fractions integration calculator. Our calculator also evaluates the antiderivative function where the degree of the numerator is higher than the degree of the denominator expression.
What is the Integration by Parts?
Integration by parts is defined as the method of integration used when two different functions are multiplied. It is used when the integrals are difficult to evaluate from the substitution method.
Integration by parts is often used to integrate products of functions involving polynomials, exponential functions, logarithmic functions, trigonometric functions, and other types of functions. It is a fundamental technique in calculus and is widely applied in various fields such as mathematics, physics, engineering, and economics.
Additionally, Integration by parts is a used in calculus to evaluate the integral of a product of two functions. Further, if you would like to find the solution of integral problems that cannot be solved by any other method of integration. you can use our trigonometric substitution calculator. Our calculator also help you solve integrals more efficiently and accurately.
Formula used by Integral by Parts Calculator:
The formula of integration by parts used by integration by parts calculator contains functions. Integration by parts formula has two product functions.
$$ \int u.v dx \;=\; u \int v dx - \int \Biggr[\frac{du}{dx} \Biggr(\int v dx \Biggr) \Biggr] dx $$
where,
u is the first function
v is the second function.
dx is the integration variable.
In this formula, we select one function, u to be differentiated and the other function, v, to be integrated. By applying the integration by parts formula, the original integral is transformed into a simpler form that can be more easily evaluated.
Additionally, For assistance with selecting appropriate functions u and v, you can utilize our u substitution integration calculator. This tool helps simplify integrals by substituting variables and functions, making complex integrals more manageable.
How Does an Integrate By Parts Calculator Work?
The integration by parts solver is simply designed to give complex problem results related to integration by part method. When you enter an integral function in the calculator whether it is definite or indefinite it starts to analyze the given function structure. Based on the problem format we decide whether u is the first function orv is the second function using the ILATE rule for integration by parts.
In ILATE rule inverse, logarithmic, algebraic, trigonometry, exponential function as a first function. After that, it takes the first function integration and treats the second as constant then separates it into a minus sign along with an integral sign as it has first function integration and takes the derivative of the second function as per the formula.
In this way, the calculation is going on till the integral function is reduced to the simplest form with constant C. In the case of definite after integration, it applies the upper and the lower limits respectively.
Related: For quick and accurate computation of definite integrals, you can also try our integral definite calculator.
Moreover, results obtained by our online integration by parts calculator with steps are precise because it works automatically as it simplifies the process which reduces the risk of mistakes. With that, it offers you detailed explanations that you can use to get a deep knowledge of the integration method. Let's take an example of the integral function solutions.
Example of the Integral By Parts
Now you see an example of a definite integral function,
Example:
$$ \int_{0}^{2} ( xe^x ) dx $$
Solution:
The definite integrals are,
$$ \int_{0}^{2} x e^x dx \;=\; 1 + e^2 \approx 8.3891 $$
The steps are as follows,
First of all, calculate the definite integral,
$$ \int_{0}^{2} e^x xdx $$
For the integrand ex, integrate by parts, ∫fdg = fg - ∫gdf, where
$$ f \;=\; x, dg \;=\; e^x dx $$
$$ df \;=\; dx, g \;=\; e^x $$
$$ e^x x|_{0}^{2} - \int_{0}^{2} e^x dx $$
Evaluate the antiderivative at the limits and subtract,
$$ e^x x \Biggr|_{0}^{2} \;=\; e^2 2 - e^0 0 \;=\; 2e^2 $$
$$ 2e^2 - \int_{0}^{2} e^x dx $$
By applying the fundamental theorem of calculus, the antiderivative of ex,
$$ 2e^2 + (-e^x) \Biggr|_{0}^{2} $$
Evaluating the antiderivative at the limits and subtracting,
$$ (-e^x) \Biggr|_{0}^{2} \;=\; (-e^2) - (-e^0) \;=\; 1 - e^2 $$
So, the answer is,
$$ =\; 1 + e^2 $$
Thus it is the final solution of our function with specific limits. If you would like to solve the indefinite integral of the given solution, you can use our calculator indefinite integral. Our calculator also helps to find the indefinite integral of the indefinite function.
How to Use the Integration by Parts Calculator?
Integrate by parts calculator is used to solve difficult antiderivative problems effortlessly when you utilize it as if you follow some simple steps. These are:
Step 1:
Enter the function in the format of ∫uv in the input field
Step 2:
Choose thevariable of integration
Step 3:
Select the type of integral from definite to indefinite
Step 4:
If you select definite then add the upper and lower limit value
Step 5:
Review your given function before hitting the calculate button.
Step 6:
Press the “Calculate” button to get the solution of the given integral function.
For additional assistance with antiderivative problems or to explore more antiderivative functions, you can utilize our finding antiderivatives calculator. Our calculator also help to find the solution of an antiderivative function whether it belongs to the definite or indefinite type.
Results Obtained from ibp Calculator
Our integration by parts calculator with steps will give you results with a few extra options for more clarity on this concept. These options are:
Additionally, for a broader range of calculators covering various mathematical concepts and problem-solving tools, explore our All Calculator page.
- Result of given function along with input function.
- Click on possible steps to get a solution with steps.
- Click Recalculate” button for further results of integral by parts problems.
Advantages of Using Integration by Parts Solver
The by parts integration calculator is an amazing tool that gives tons of benefits. With that, it gives you proper assistance in solving the integrands of your given function; you do not need to put any external effort into complex functions. These benefits are:
The integral by parts calculator can evaluate different types of functions whether it is definite or indefinite integrals.
It is a reliable tool, as it saves time from doing lengthy calculations
Our integrate by parts calculator provides results in a few seconds.
It has a friendly interface, and everyone can easily access it.
You can use our integral calculator by parts for practice by giving any type of function as an example.
Our integration by parts calculator can manage various types of integral functions.
Related:Need assistance with other integration methods? you can use our integration solve online that offers additional support for various integral types.