Introduction to Area Under the Curve Calculator
The area under a curve calculator is an integral tool that is used to find the Area under a bounded region. It evaluates the integral function which is enclosed under a graph that is lying below or above the curve of the x-axis in the run of time.

So arae under the curve calculator help you to solve area under a bounded region. Additionally,if you need to evaluating bounded areas accurately. You can utilize our definite integral calculator.This is particularly useful in calculus for determining accumulated quantities such as total distance traveled or total work done.
What is the Area Under the Curve?
The area under the curve is defined as a definite integral function in a bounded above or below region along the x-axis. It uses integration rules to find the Area under the curve and sketch a graph that tells the area is present above or below the x-axis. It represents the total accumulation of quantities such as distance, volume, or other measurable values, as depicted by the curve.
Additionally, When we have a single-variable function f(x) and we want to find the area under its curve between two points a and b, we can use a definite integral. Furthermore, when we have a two-variable function f(x,y) defined over a region in the xy-plane, you can use our double integral calculator to find the volume under the surface represented by the function within that region.
Formula Used by Area Under Curve Calculator
The area under the curve formula is based on the definite integral. The notation used by the area under the curve calculator is,
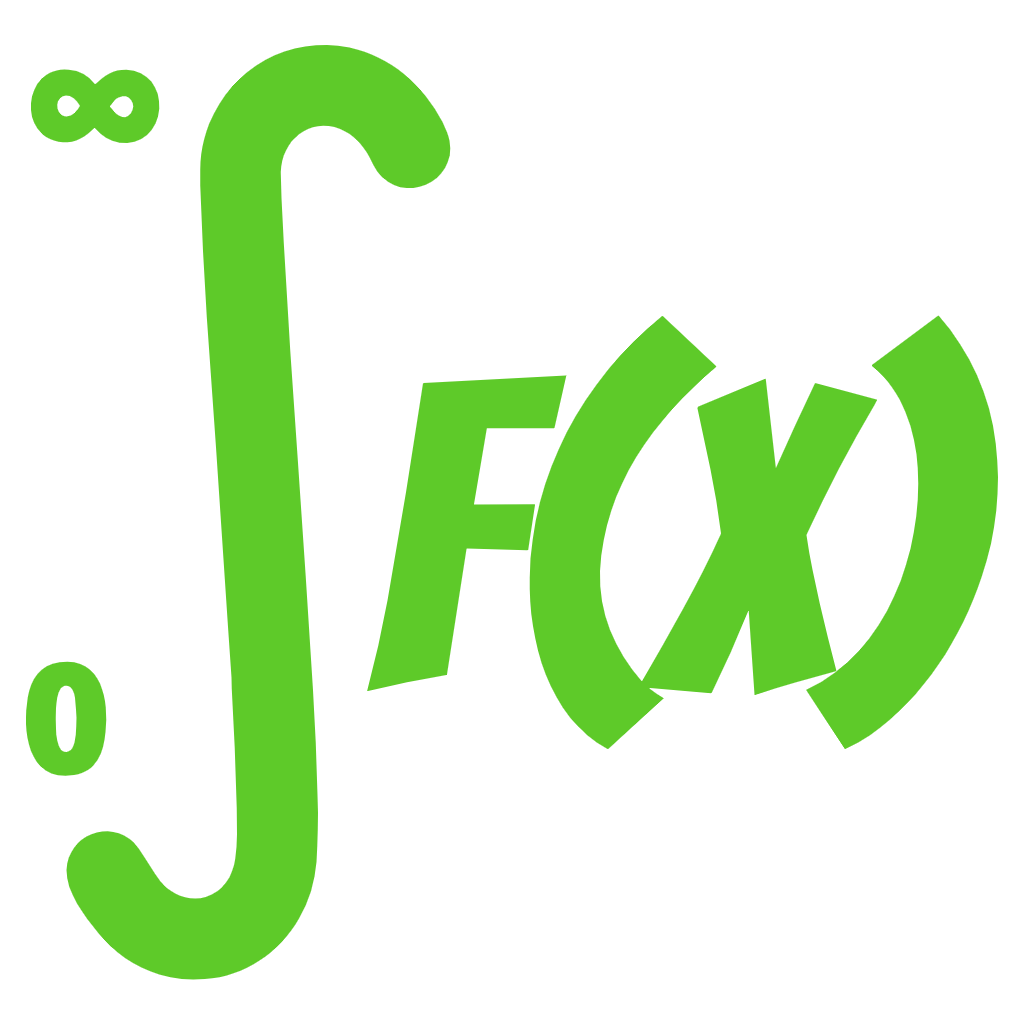
$$ Area\;=\; \int_{a}^{b} f(x) dx \;=\; F(b) - F(a) $$
Where,
For computations involving line integrals, you can also use our Line Integral Calculator for efficient and precise solutions.It simplifies the computation of integrals along curves in vector fields, aiding in various applications in physics, engineering, and more.
- A= area under the curve
- a,b is the upper or lower limit.
- f(x) is the integral function along the x-axis
- dx is an integral variable
Working Method of the Area Under a Curve Calculator
The area under curve calculator works on the principle of integration to solve the area under the curve. You just add your required function to evaluate the area of the graph in the calculator and get a solution instantly.
When you enter your integral function in the area under graph calculator, it will check the attribute of the given function whether it is a definite or indefinite problem. Then apply the integral rule to your particular function for integration.
After integration, the graph area calculator applies both the upper and lower limits and you get the solution of the area under the curve. In this way, you can find any type of graph in this calculator without any inconvenience.
After that calculator displays the result, providing the area under the curve based on the input function and any specified bounds.If you're interested in calculate triple integrals, you can use our Triple Integral Calculator for efficient and accurate solutions. Triple integrals extend the concept of integration to three dimensions, enabling the calculation of volumes and other quantities within three-dimensional space.
Now let's examine an example of an area under the curve in our area under the graph calculator to see its works with the visual lens.
Example of the Area of Curve
The area under the curve can be determined using the Area under the curve calculator but it’s crucial to know each step thoroughly. Here’s an example to help you understand each step,
Example:
Find the area under the curve of:
$$ f(x) \;=\; 4-x^2 \;from\;x \;=\; -2 \;to\; x\;=\;2 $$
Solution:
$$ Area\;=\; \int_{-2}^{2} (4-x^2) dx $$
Evaluate the antiderivative of f(x) from x= -2 and x=2.
$$ \int (4-x^2) dx \;=\; \int 4 dx - \int x^2 dx $$
$$ 4x - \frac{x^{2+1}}{2+1} + C $$
$$ 4x - \frac{x^3}{3} + C $$
$$ Area\;=\; \biggr[ 4x - \frac{x^3}{3} \biggr]_{-2}^{2} $$
$$ \biggr[ 4(2) - \frac{2^3}{3} \biggr] - \biggr[ 4(-2) - \frac{(-2)^3}{3} \biggr] $$
$$ \frac{32}{3} $$
The graph of the above calculation is given as,
“PUT THE GRAPH HERE”
Thus it is the final solution of our function with specific limits. Further, if you wish to handle both indefinite or definite integrals, our antiderivative calculator will evaluate any antidifferentiation function. You only need to input the function and the integration bounds to get the result.
How to Use the Area Under the Curve Calculator
The integral area calculator evaluates the area enclosed under a curve in simple steps you just need to follow our instructions. These instructions are:
Additionally, our calculator always gives you accurate results of a given area of the integral function. Further, If you're looking to find antiderivatives without specified limits, check out our indefinite integral calculator for more flexibility.
- Enter the required integral function in the input box
- Add upper and lower limits in its box (if a given function is the definite integral
- For indefinite integral function, you do not need to put any limit value in the area under curve calculator.
- Click on the “Calculate” button to get the solution of the area under the curve.
Result Obtained from Area Under Graph Calculator
You will get the result in a fraction of a second when you add an input function in the area under a curve calculator. It may include as:
- Result
It will give you the result of your given integral function immediately
- Possible steps
It gives results in step by step-by-step process for a better understanding
- Recalculate
You will get a new page for more calculations to find the area.
For calculations involving improper integrals, you may use an improper integral calculator to simplify the process. It handles integrals with infinite limits or discontinuous integrands efficiently, providing accurate solutions for complex mathematical scenarios.
Advantages of Integral Area Calculator
An area under the graph calculator provides you with tons of benefits while you are doing calculations of the area under the curve problems. These advantages are,
Overall, the Area under the Curve Calculator serves as a valuable tool for researchers, students, and professionals alike, facilitating accurate and efficient computation of enclosed areas in mathematical and scientific analyses. For more comprehensive mathematical computations, you can also utilize our Integral Calculator, which offers solutions for both indefinite and definite integrals, enhancing problem-solving capabilities across various disciplines.
- It saves the time that you consume in doing long-form calculations of the area under the curve
- Area under the curve Calculator is a free tool, you can operate it anywhere through internet connectivity
- It has a simple interface so everyone can easily use it.
- The area under graph calculator always gives you accurate results of a given area of the integral function
- It keeps you away from trouble from doing long-form calculations.